回复
这个树,怎么一下就平衡了?(三)
joytrian
发布于 2022-7-6 17:06
浏览
0收藏
代码实现
首先对于节点多个height属性。用于计算高度(平衡因子)
插入是递归插入,递归是一个来回的过程,去的过程进行插入,回的过程进行高度更新,和检查是否平衡。推荐不要写全局递归计算高度,效率太低下,事实上高度变化只和插入和平衡有关,仔细考虑即不会有疏漏!
代码写的比较早,如有命名不规范的情况,还请勿喷,如果有疏漏还请指出!
import java.util.ArrayDeque;
import java.util.Queue;
public class AVLTree {
class node
{
int value;
node left;
node right;
int height;
public node() {
}
public node(int value)
{
this.value=value;
this.height=0;
}
public node(int value,node left,node right)
{
this.value=value;
this.left=left;this.right=right;
this.height=0;
}
}
node root;// 根
public AVLTree() {
this.root = null;
}
public boolean isContains(int x)// 是否存在
{
node current = root;
if (root == null) {
return false;
}
while (current.value != x && current != null) {
if (x < current.value) {
current = current.left;
}
if (x > current.value) {
current = current.right;
}
if (current == null) {
return false;
} // 在里面判断如果超直接返回
}
// 如果在这个位置判断是否为空会导致current.value不存在报错
if (current.value == x) {
return true;
}
return false;
}
public int getHeight(node t)
{
if(t==null) {return -1;}//
return t.height;
//return 1+Math.max(getHeight(t.left), getHeight(t.right));这种效率太低
}
public void cengxu(node t) {//层序遍历
Queue<node> q1 = new ArrayDeque<node>();
if (t == null)
return;
if (t != null) {
q1.add(t);
}
while (!q1.isEmpty()) {
node t1 = q1.poll();
if (t1.left != null)
q1.add(t1.left);
if (t1.right != null)
q1.add(t1.right);
System.out.print(t1.value + " ");
}
System.out.println();
}
public void zhongxu(node t)//中序遍历 中序遍历:左子树---> 根结点 ---> 右子树
{//为了测试改成中后都行
if(t!=null)
{
zhongxu(t.left);
System.out.print(t.value+" ");//访问完左节点访问当前节点
zhongxu(t.right);
//System.out.print(t.value+" ");//访问完左节点访问当前节点
}
}
public void qianxu(node t)//前序递归 前序遍历:根结点 ---> 左子树 ---> 右子树
{
if(t!=null) {
System.out.print(t.value+" ");//当前节点
qianxu(t.left );
qianxu(t.right);}
}
public void insert(int value) {
root=insert(value, root);
}
public node insert(int x,node t)//插入 t是root的引用
{
node a1=new node(x);
//if(root==null) {root=a1;return root;}
if(t==null) {return a1;}
//插入操作。递归实现
else if(t!=null)
{
if(x<t.value)
{ t.left=insert(x,t.left);}
else
{ t.right= insert(x,t.right);}
}
/*
* 更新当前节点的高度,因为整个插入只有被插入的一方有影响,
* 所以递归会更新好最底层的,上层可直接调用
*/
t.height=Math.max(getHeight(t.left),getHeight(t.right))+1;//不要写成递归, 递归效率低
return banlance(t);//因为java对象传参机制,需要克隆,不可直接t=xx 否则变换
}
private node banlance(node t) {
// TODO Auto-generated method stub
//if(t==null)return null;
int lefthigh=getHeight(t.left);
int righthigh=getHeight(t.right);
if(Math.abs(lefthigh-righthigh)<=1)//不需要平衡滴
{ return t;}
else if(lefthigh<righthigh)//右侧大
{
if(getHeight(t.right.left)<getHeight(t.right.right))//RR需要左旋
{
return getRRbanlance(t);
}
else {
return getRLbanlance(t);
}
}
else {
if(getHeight(t.left.left)>getHeight(t.left.right))//ll 左左
{
return getLLbanlance(t);
}
else {
return getLRbanlance(t);
}
}
}
/*
* oldroot(平衡因子为2,不平衡) ==> newroot
* / \ / \
* B newroot(平衡因子为1) oldroot D
* / \ / \ \
* C D B C E
* \
* E
*/
private node getRRbanlance(node oldroot) {//右右深,需要左旋
// TODO Auto-generated method stub
node newroot=oldroot.right;
oldroot.right=newroot.left;
newroot.left=oldroot;
oldroot.height=Math.max(getHeight(oldroot.left),getHeight(oldroot.right))+1;
newroot.height=Math.max(getHeight(newroot.left),getHeight(newroot.right))+1;//原来的root的高度需要从新计算
return newroot;
}
/*
* 右旋同理
*/
private node getLLbanlance(node oldroot) {//LL小,需要右旋转
// TODO Auto-generated method stub
node newroot=oldroot.left;
oldroot.left=newroot.right;
newroot.right=oldroot;
oldroot.height=Math.max(getHeight(oldroot.left),getHeight(oldroot.right))+1;
newroot.height=Math.max(getHeight(newroot.left),getHeight(newroot.right))+1;//原来的root的高度需要从新金酸
return newroot;
}
private node getLRbanlance(node oldroot) {
oldroot.left =getRRbanlance(oldroot.left);
oldroot.height=Math.max(getHeight(oldroot.left), getHeight(oldroot.right))+1;
return getLLbanlance(oldroot);
}
/* (不平衡出现在右左节点)
* oldroot ==> newroot
* / \ / \
* A B oldroot B
* / \ / \ / \
* newroot D A E F D
* / \
* E F
*/
private node getRLbanlance(node oldroot) {//右左深
// node newroot=oldroot.right.left;
// oldroot.right.left=newroot.right;
// newroot.right=oldroot.right;
// oldroot.right=newroot.left;
// newroot.left=oldroot;
// oldroot.height=Math.max(getHeight(oldroot.left),getHeight(oldroot.right))+1;
// newroot.right.height=Math.max(getHeight(newroot.right.left),getHeight(newroot.right.right))+1;
// newroot.height=Math.max(getHeight(oldroot.left),getHeight(newroot.right))+1;//原来的root的高度需要从新金酸
oldroot.right =getLLbanlance(oldroot.right);
oldroot.height=Math.max(getHeight(oldroot.left), getHeight(oldroot.right))+1;
return getRRbanlance(oldroot);
}
}
测试情况:
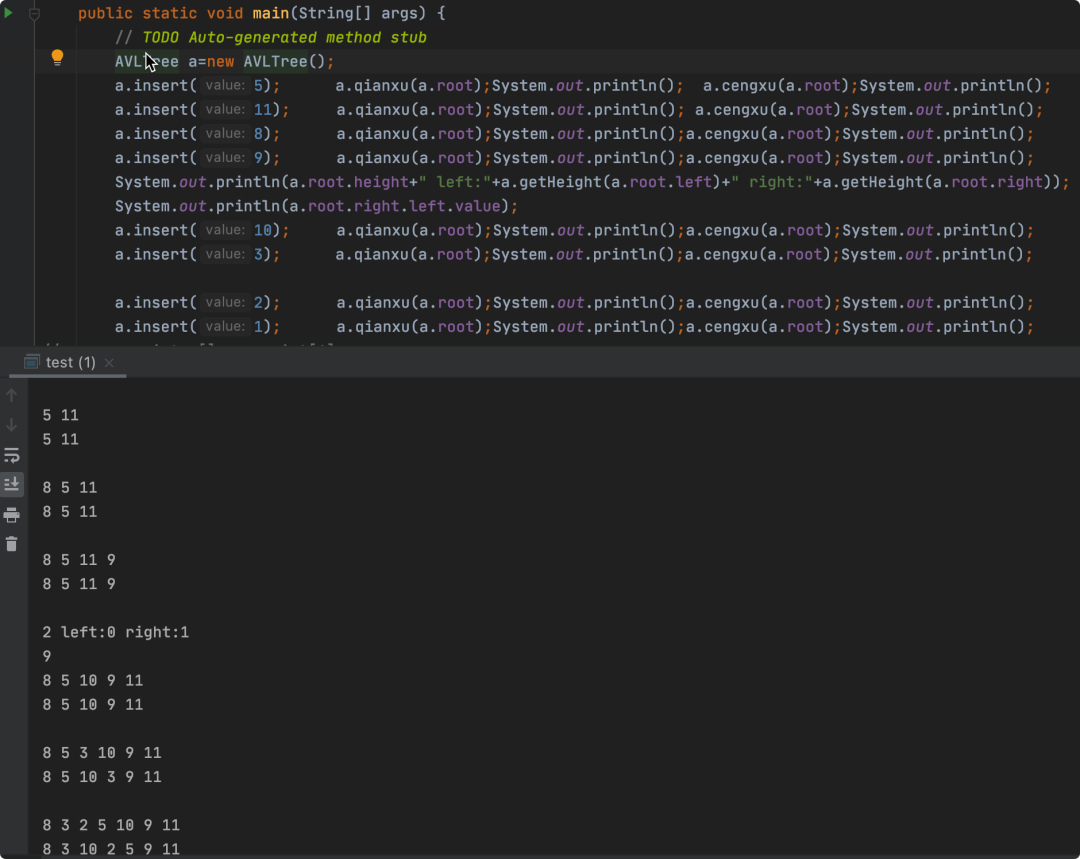
AVL的理解需要时间,当然笔者的AVL自己写的可能有些疏漏,如果有问题还请各位一起探讨!
当然,除了插入,AVL还有删除等其他操作,(原理相似。删除后平衡)有兴趣可以一起研究。
结语
原创不易,如果本文对你有帮助,还请动动小手,帮忙点个赞和在看,分享给好友或者朋友圈,谢谢啦!
文章转自公众号:bigsai
标签
已于2022-7-6 17:06:01修改
赞
收藏
回复
相关推荐



















